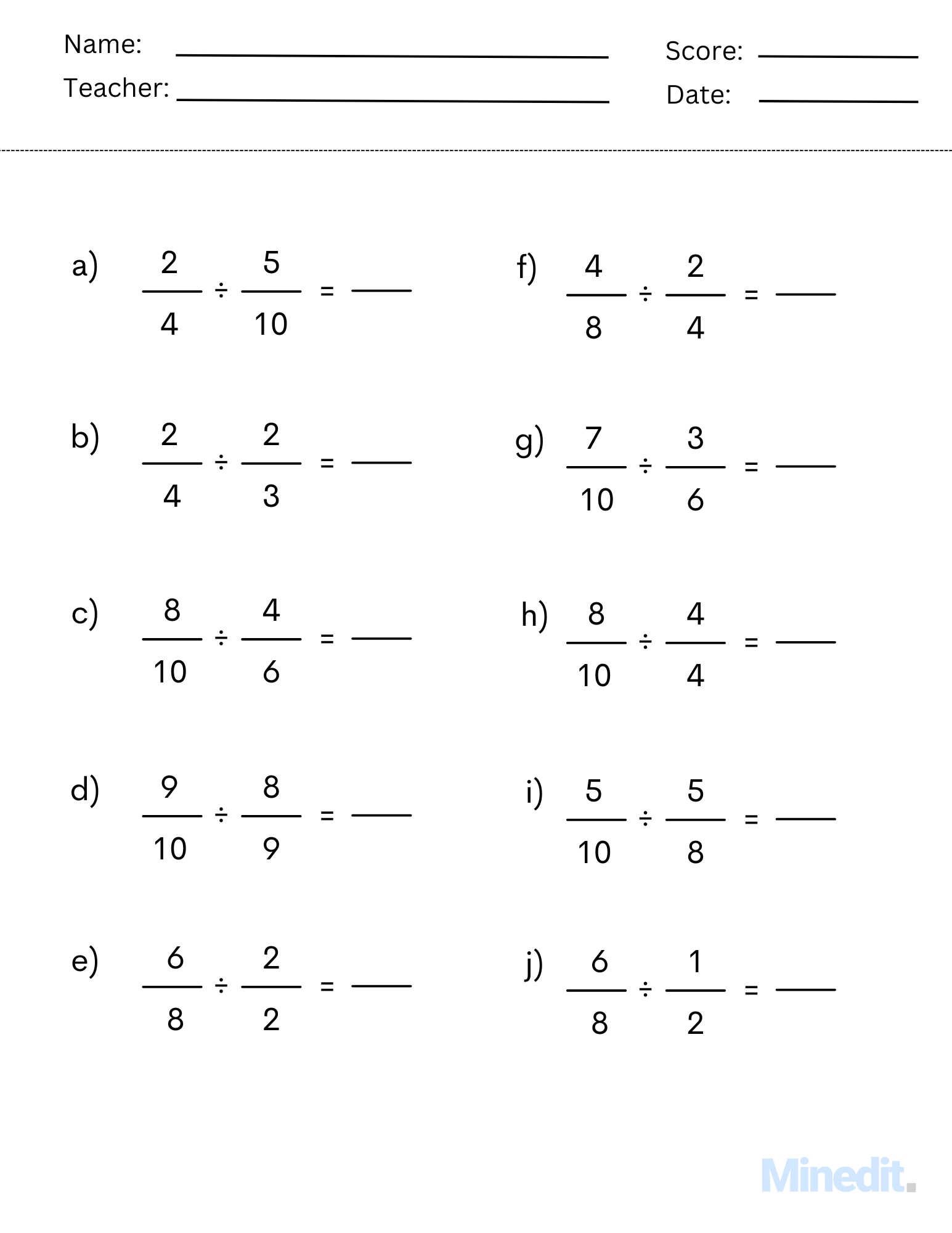
dfw_1
DownloadDividing fractions is one of those math concepts that can initially seem challenging for many students. However, once you grasp the fundamental rules and practice enough, it becomes much simpler and even enjoyable! One of the most effective ways to practice dividing fractions is by using a Dividing Fractions Worksheet. Whether you’re a student working on homework, a teacher looking for classroom resources, or a parent trying to help your child, dividing fractions worksheets are essential tools for mastering this concept.
In this blog post, we will take an in-depth look at dividing fractions, including the rules and steps involved, why this skill is important, and how a dividing fractions worksheet can help reinforce understanding. We’ll also explore some common mistakes to avoid, and offer a few tips and tricks to make learning fractions easier.
What Are Fractions?
Before diving into how to divide fractions, let’s briefly revisit what a fraction is. A fraction represents a part of a whole and consists of two numbers:
- The Numerator: The top number of a fraction, which indicates how many parts we are talking about.
- The Denominator: The bottom number of a fraction, which indicates the total number of parts in the whole.
For example, in the fraction 3/4, the numerator (3) tells us we have 3 parts, and the denominator (4) tells us that the whole is divided into 4 equal parts. In simple terms, 3/4 means “3 out of 4 equal parts.”
What Does It Mean to Divide Fractions?
Dividing fractions essentially means finding out how many times one fraction fits into another. While the concept may sound complex, the operation itself becomes simple once you understand the key rule: to divide fractions, you multiply by the reciprocal.
In other words, when dividing one fraction by another, you:
- Flip the second fraction (the divisor) to create its reciprocal.
- Multiply the first fraction (the dividend) by the reciprocal of the second.
Here’s a step-by-step breakdown of how to divide fractions:
Steps to Divide Fractions
Dividing fractions might sound complicated, but once you break it down, it’s a straightforward process. Follow these simple steps:
Step 1: Write the Reciprocal of the Divisor
The reciprocal of a fraction is created by swapping its numerator and denominator. For example, the reciprocal of 3/5 is 5/3. When dividing fractions, the first thing you do is take the reciprocal of the second fraction (the divisor).
Step 2: Change the Division to Multiplication
Once you’ve found the reciprocal of the divisor, change the division operation to multiplication. So instead of dividing by the second fraction, you’re now multiplying by its reciprocal.
Step 3: Multiply the Numerators
Now, multiply the numerators (the top numbers) of both fractions to get the numerator of your answer.
Step 4: Multiply the Denominators
Next, multiply the denominators (the bottom numbers) of both fractions to get the denominator of your answer.
Step 5: Simplify the Fraction (if needed)
Lastly, if possible, simplify the fraction by finding the greatest common factor (GCF) of the numerator and denominator and dividing both by it. If the result is an improper fraction, you might also convert it into a mixed number.
Example of Dividing Fractions
Let’s walk through an example problem to see how these steps work in practice.
Example: Divide 2/3 by 4/5.
- Find the reciprocal of the divisor (4/5): The reciprocal of 4/5 is 5/4.
- Change the division to multiplication: Instead of 2/3 ÷ 4/5, the problem becomes 2/3 × 5/4.
- Multiply the numerators: 2 × 5 = 10.
- Multiply the denominators: 3 × 4 = 12.
- Simplify: The fraction 10/12 can be simplified by dividing both the numerator and the denominator by 2, giving us 5/6.
So, 2/3 ÷ 4/5 = 5/6.
Why Is Dividing Fractions Important?
Dividing fractions may seem like a specific skill, but it’s an essential part of understanding how numbers and mathematical operations work. Here are a few reasons why mastering this skill is important:
1. Real-Life Applications
Dividing fractions comes in handy in everyday life. Whether you’re splitting a recipe in half, dividing up portions of food, or working with measurements in construction, the ability to divide fractions makes many practical tasks easier. For example, if a recipe calls for 2/3 cup of sugar, but you only want to make half of the recipe, you’ll need to divide 2/3 by 2, which means multiplying 2/3 by the reciprocal of 2, or 1/2. This gives you 2/6, which simplifies to 1/3. So, you would use 1/3 cup of sugar in your smaller recipe.
2. Foundation for Advanced Math
Dividing fractions is not only a useful skill in daily life but also a foundational skill for more advanced math topics, including algebra, calculus, and trigonometry. When working with variables and equations in algebra, you’ll often encounter situations where dividing fractions is necessary to solve problems. Mastering this concept now prepares students for success in higher-level math courses.
3. Improves Number Sense
Understanding how fractions work, including how to divide them, helps students develop better number sense. Number sense is the ability to understand, interpret, and work with numbers in various forms. By dividing fractions, students gain a deeper understanding of proportionality and how different numbers relate to one another.
Why Use a Dividing Fractions Worksheet?
Worksheets are valuable tools in any math classroom or for home practice. Here are some reasons why using a Dividing Fractions Worksheet is an effective way to master this important skill:
1. Structured Practice
Worksheets provide a structured way to practice dividing fractions. They often include a range of problems, from simple to more complex, allowing students to build their skills gradually. A fantastic worksheet will cover various scenarios, including problems with whole numbers, improper fractions, and mixed numbers.
2. Reinforcement
Repetition is key when learning a fresh math concept, and dividing fractions is no exception. A worksheet provides the opportunity to repeat the process of dividing fractions multiple times, helping to reinforce the steps and improve accuracy.
3. Confidence Building
For students who may feel intimidated by fractions, a worksheet offers a way to build confidence. As they work through the problems and see that they can successfully divide fractions, their confidence in their math abilities grows. Worksheets can help demystify the process and show students that they are capable of tackling even the most challenging math problems.
4. Immediate Feedback
When teachers or parents use worksheets, they can quickly review a student’s work and provide feedback. This immediate feedback allows students to correct their mistakes and understand where they went wrong, helping to prevent the same errors in the future.
5. Engaging and Interactive
Many worksheets are designed to be engaging, offering students a chance to interact with the material in a hands-on way. Some worksheets might include visual aids, word problems, or puzzles that make learning more fun and less repetitive.
Common Mistakes to Avoid When Dividing Fractions
While dividing fractions becomes simple with practice, students often make some common mistakes along the way. Here’s what to look out for:
1. Forgetting to Find the Reciprocal
One of the most common mistakes is forgetting to flip the second fraction before multiplying. Without this crucial step, the division problem becomes a multiplication problem with the wrong fractions, leading to an incorrect answer.
2. Incorrect Multiplication of Numerators or Denominators
Sometimes students multiply the numerator of one fraction by the denominator of the other, mixing up the multiplication process. Always remember to multiply the numerators together and the denominators together.
3. Not Simplifying the Final Answer
While it’s not always necessary, simplifying the fraction (if possible) helps students arrive at the most accurate and clean answer. Failing to simplify can result in a correct answer, but one that’s harder to interpret.
4. Confusion with Mixed Numbers
When dividing mixed numbers, students must first convert them to improper fractions before dividing. Trying to divide mixed numbers without converting them leads to confusion and incorrect results.
Tips and Tricks for Success with Dividing Fractions
To help you master dividing fractions, here are some helpful tips and tricks:
1. Practice with Worksheets
The best way to get comfortable with dividing fractions is through regular practice. A Dividing Fractions Worksheetwill give you the repetition needed to solidify your understanding and ensure that you remember the steps.
2. Double-Check Your Work
Always go back and double-check your work, especially your multiplication. Double-check that you’ve correctly flipped the second fraction and that your final answer is simplified if necessary.
3. Use Visual Aids
For visual learners, using fraction bars or drawing models can help make sense of dividing fractions. Visual aids allow students to see how fractions are divided in a more concrete way.
4. Don’t Be Afraid to Make Mistakes
Mistakes are part of learning. If you get an answer wrong, try to understand where you went wrong and correct your mistake. Learning from errors is one of the best ways to improve.
Conclusion
Dividing fractions is a key skill that every math student must master. While it can initially seem tricky, following the right steps and practicing regularly can make it much easier. A Dividing Fractions Worksheet is an excellent tool for students to practice dividing fractions in a structured and engaging way. By breaking down the process, practicing regularly, and avoiding common mistakes, students will develop confidence and proficiency in this important math skill.
Whether you’re a student looking to improve your math abilities, a teacher preparing resources for the classroom, or a parent helping your child with homework, dividing fractions worksheets are a valuable resource to help reinforce learning and ensure success. With enough practice, dividing fractions can become second nature, preparing students for more advanced math concepts in the future! Thanks for visiting Minedit!